|
|
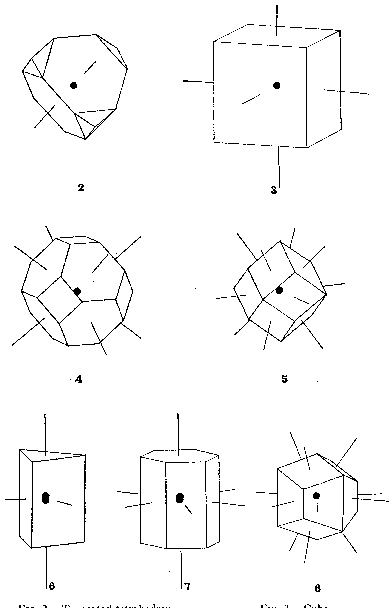
Volume 9, pages 45-54, 1924 EDGAR T. WHERRY, Bureau of Chemistry, Washington, D. C. As a solution cools down, or the solvent is removed by evaporation, a point is ultimately reached at which material separates in solid form. The first molecule to deposit attracts others to itself and a solid mass is gradually built up. If the attractive forces are without any particular orientation in space, the arrangement will be a random one, and the material is classed as amorphous. In most cases, however, the forces are definitely oriented and the incoming molecules are attracted in four or more directions, so as to take up characteristic arrangements in space, the solid then being said to have a crystalline structure. THE POLYHEDRAL DOMAIN VIEWPOINT. --- In working out crystal structures by X-ray measurements and other methods, the positions of the centers of the molecules are usually considered, thus greatly simplifying the mathematical treatment of the subject. The visualization of the relations between the structure and the attractive forces concerned and the appreciation of the significance of many of the peculiar phenomena shown by crystals become much easier, however, when use is made of the molecular "spheres of influence" or domains. The customary assumption is that the domains are spherical but were such actually the case close-packed arrangements would be expected to be normal and common, whereas the X-ray studies thus far made indicate close-packing to be exceptional and infrequent. The domains are therefore regarded here, as they were long ago by Hauy, the great pioneer in the development of crystallography, as having polyhedral shapes, with the principal faces perpendicular to the main lines of concentration of the attractive forces. Figure 1 shows the relation between a point-system and the shape of the corresponding domain. The principal types of domains represented among simple crystals, in many of which the "molecules" consist of single atoms, may well be figured at this point. Most of these, it should be noted, are different in shape from Hauy's "integrant molecules," for he admitted several which were not capable of solidly filling space, whereas all here shown can do so. Distorted and modified representatives of them probably underlie many sorts of crystals. A given kind of atom (or molecule) does not always exhibit the same shape of domain. Carbon atoms occupy truncated tetrahedrons in diamond, but trigonal prisms (apparently somewhat modified by dissimilar pyramids at the opposite ends) in graphite, distorted cubes in calcite, and still other forms in other compounds. Sodium atoms occupy truncated octahedrons in the metal itself, cubes more or less bevelled by dodecahedron faces in halides, and cubes bevelled by other forms in modified crystals of some of its compounds. But variation in this respect is not regarded as rendering the conception of polyhedral domains valueless; on the contrary, a study of the conditions bringing about the shift from one domain shape to another seems adapted to throw light on the whole subject of atomic attraction.
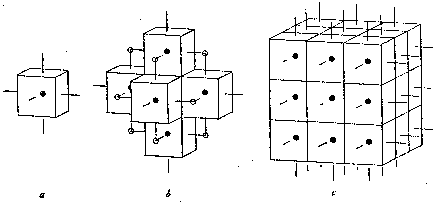
THE DOMAIN-LAYER CONCEPTION OF CRYSTAL BUILDING. - The principal faces of the domains are regarded as perpendicular to the force lines along which atoms or molecules are attracted to one another. When a crystal builds up from domains the surface form will tend to be such that the minimum number of these force lines is free. Thus in the case of cubic domains, as shown in figure 9, the original cube, a, first attracts others to it to yield arrangement b. Now, however, each step-depression has two projecting force lines, so that they will fill up before more domains attach themselves to the surfaces of the outer cubes, where but single force-lines project. The corner-depressions thereby formed, having three lines each, will be still more readily filled up, the result being the larger cube, c.
The same general process should be followed, no matter how large the crystal becomes, one layer of molecules (or atoms) being completed before another begins. It is interesting to note that in the electrolytic deposition of crystals of metals, as followed under the microscope, this sort of growth can be actually seen to take place, since the layers, instead of being but one molecule in thickness, are of visible dimensions. 2
FREE FORCE LINES.-While the building up of crystals from domains proceeds in such a manner that the number of free force lines is reduced to a minimum, the final surface of every crystal must always have numerous such lines extending up from it, ready to seize upon other atoms which come their way. Herein lies the origin of the interesting phenomena of definitely oriented growth of one crystal on another as observed in natural minerals, arid in salts caused to crystallize, or liquid crystals allowed to develop, on the cleavage surfaces of crystals. These have been described by a number of recent workers, and will not be further discussed here. THE CONCEPTION OF NORMAL FORM-COMBINATION. - The packing together of cubes is especially easy to visualize. To bring out the relations in certain other kinds of domains, Bristol-board models have been constructed and packed together, the results being illustrated in figure 10, a to c. This plan may be recommended to anyone desiring to obtain a clear idea of the significance of the relations discussed in this article. Inspection of such assemblages will bring out one additional feature: the surface configuration showing the minimum number of free force lines (exposed domain faces) may be different from that of the domain. itself. Thus, as pointed out by Fedorov, 3 truncated octahedral domains will yield rhomb-dodecahedral crystals, and rhomb-dodecahedral domains simple octahedral crystals. But whether domain and final solid are like or unlike in shape, if in the course of crystal building access of material were uniform on all sides, and there were no interference from external sources, the final result would be a symmetrically developed polyhedron of a shape or form-combination characteristic for each domain type.
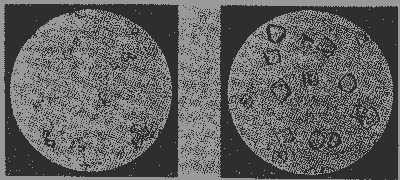
In reality these conditions are practically unattainable, and more or less deviation from the theoretical symmetry is nearly if not quite universal. Layers of domains often deposit around the original nucleus in greater abundance on some surfaces than on others, even though every layer is in itself complete, the interfacial angles, however, remaining normal. This "form distortion" of crystals is so well known that it needs no further discussion; its origin is external and it does not conflict with the general principle that every type of domain tends to yield, when built up into a crystal, a definite, normal form-combination. DEVIATIONS FROM THE NORMAL FORM-COMBINATION. - As expressed by Hauy long ago in his "law of decrements," deviations from the normal form-combination of a crystal are connected with the decreasing width of successive domain-layers. The relations between the number of domains in the opposing sides of the depressions thus produced will determine the slopes and crystallographic symbols of the resulting faces; when the ratios are small the new face will have simple indices, but when they are larger, the indices will become complex, and ultimately the faces will be vicinal ones. Hauy is not known to have suggested any definite mechanisms which might cause decrements in domain layers; nor do any subsequent students appear to have done so. 4 In the following two paragraphs an effort is made to account for decrements in a simple manner.VICINAL FACES AS THE RESULT OF SURFACE TENSION. - Angle distortion, or the development of vicinal faces, may at times, like form-distortion, be external in origin, and connected with the mode of approach of the saturated solution to the surface of the growing crystal. It is believed, however, to be more frequently internal in the sense of representing a balance between the directed attractive forces of the individual domains and the general effect of massed domains known as surface tension. This latter force tends to make a mass of material spherical, and in the case of liquid drops it succeeds; but in crystals it can not go so far. The multiplication of lines of attractive force in depressions leads, as already shown, to a tendency on the part of domain-layers to fill out completely. But the influence of surface tension is toward the prevention of such complete filling; and when the two tendencies balance, an equilibrium or vicinal form becomes stable. In the mineral fluorite, for example, the cube is often replaced by a vicinal tetrahexahedron (ζ) with an angle of slope of 1° 48', corresponding to the symbol (32.1.0), or, from the point of view here held, to each successive domain layer being 32 domains shorter at its margins than the next deeper one. Since surface tension is ever present, the development of such vicinal faces is to be looked upon as a normal phenomenon of crystals, and the slopes of these faces as being not chance matters, but definite expressions of forces inherent in and characteristic of each substance. MODIFICATION AS THE RESULT OF THE PRESENCE OF IMPURITIES. - The steps between domain layers represented by vicinal faces have a considerable number of domains on one side to a single one on the other. Steps with more nearly equal sides are, however, still more frequently developed, and corresponding modifying forms appear on the edges of the normal form-combination corresponding to the domain, or bound the crystal completely. The most satisfactory illustration of this should be a compound that is relatively simple in structure, that can be crystallized readily, and that responds markedly to changes in external conditions, requirements well met by sodium chloride. Examination of its crystals by X -rays shows it to have a structure which may be regarded as a simple cubic point system, with alternate points occupied by Na and Cl atoms. The compound being a polar one, the two kinds of atoms may be treated separately. The domains of both sodium and chlorine atoms, then, are essentially cubic in shape, the edges of the cubes of the larger sodiums being merely bevelled by insignificant dodecahedron faces.As already pointed out, a crystal built up of cubical domains, if left to the sway of its own attractive forces, will be cubic in habit. That such is the relation in the case of sodium chloride can be shown by the following experiment: Place a drop of a saturated solution of NaCl in CO 2-free distilled water on a microscope slide, let stand until a ring of white solid has formed around the edge of the drop, carefully apply a cover glass, and examine under a low power microscope. The crystals which develop have a typical cubic habit, many showing form-distortion because of unequal deposition of material on different sides, but none with obvious angle distortion or modifying forms (Figure 11 a.5 To another portion of the same solution add a minute drop of dilute sodium hydroxide solution, and repeat the experiment. The crystals which form most rapidly will still be cubes, but those of slower growth will now be typical octahedrons. Depressions with three equal sides have formed at the corners of domain layers. (Figure 11 b.) To obtain a simple explanation of this phenomenon it is necessary only to assume that the attraction of sodium atoms for hydroxyl groups is concentrated along 8 instead of 6 force lines - that is, the sodium domain under these conditions tends to become octahedral. When the hydroxide is present in the sodium chloride solution, hydroxyl groups will enter the developing crystal in place of part of the chlorine atoms; but, instead of joining on at the faces of the sodium domains (as these faces are developed toward chlorine), they will tend to occupy the corners of the cubes, that is, octahedral faces. (Fig. 12.)
If the crystals grow rapidly, material may build on so fast that such minor irregularities will be swallowed up, and the final crystal habit will be the normal one. But when growth is slow, the hydroxyl groups may keep chloride domains from filling out the corners to form cubes; a sort of film of the impurity will cover the octahedral planes, which will thereby show as faces on the crystals, or even bound them completely if the quantity of impurity is sufficient. Such an "adsorbed" film could hardly prevent the further growth of a crystal covered by it, for no doubt there is continual resolution and redeposition of the uppermost layer of domains on any slowly growing crystal, permitting sodium and chlorine atoms to keep on migrating in, and to build up the interior mass in their usual way. However, the form corresponding to the film is likely to maintain itself and appear on the surface of the final crystal, though in many cases exhibiting striations which bring out the competition concerned.According to this interpretation, the more highly modified a crystal is, the greater number of adsorbable impurities were present in the solution from which it separated, although, as the extent of each modifying form is related to the quantity of the corresponding impurity and as very little is required for a layer but one atom or one group thick on a crystal of ordinary size, the total quantities of impurities necessary are small. Study of various artificially crystallizable substances with intentionally added impurities and of the form-development of individual minerals with reference to their associates at different localities should yield interesting results in this connection. ETCH-FIGURES AS SURFACE PHENOMENA. - The depressions produced on crystal faces by the momentary application of minute quantities of solvents often show a symmetry different than that of the crystal itself. Because this is sometimes due to the fact that the crystal is bounded by a general form not capable of indicating the symmetry of the structure represented, the assumption is commonly made that etch-figures always bring out the internal structure. This inference the present writer is unable to accept. These figures are regarded, instead, as the expression of the attractive forces between the atoms at the crystal surface and those of the solvent used. Their symmetry admittedly often corresponds to that of the structure; they presumably never possess a higher symmetry; but they may have a lower symmetry when the solvent acts in such a manner that the electron arrangements of the constituent atoms are brought out. In the case of sodium chloride (or, for that matter, any alkali halide) the form development of the crystals is normally holohedral, and the lack of rotation of the plane of polarized light shows conclusively that the internal structure or atomic arrangement can not be that of either of the gyroidal classes of the isometric system. X-ray study confirms the holohedral character of the structure. Nevertheless, as shown by Rosicky, etching with a strong salt solution is capable of yielding gyroidally arranged etch-figures, and they can be produced on potassium chloride even more readily. Such figures are here regarded as representing an expression of the low degree of symmetry presumably possessed by alkali metal atoms, with single surface electrons, or by halogen atoms with seven. With most solvents and many conditions of solution, the high symmetry of the structure as a whole rather than the low symmetry of the constituent elements appears to be brought out. But if the solvent at certain concentrations may be assumed to remove sodium and chlorine domains in such a manner that their individual symmetry comes into play, a simple explanation of the unquestionable difference between the symmetry of the etch figures and of the crystal structure is found. CONCLUSIONS. - Crystal structure data should be translated into a form capable of being visualized by those not skilled in advanced mathematics. The recognition of polyhedral molecular domains with lines along which the attractive forces are concentrated perpendicular to their faces makes the understanding of certain of the peculiar features of crystals relatively easy. Vicinal faces are not chance occurrences but are the result of an equilibrium of forces inherent in and characteristic of each substance. Modifying faces and even complete bounding of crystals by forms other than the normal ones developed by the undisturbed building together of the domains can be explained by the presence in the solutions of foreign constituents attracted to and depositing on the abnormal faces. Etch-figures may bring out symmetry belonging to the constituent atoms or molecules removed by the solvent, and not necessarily the structure of the crystal itself. Admittedly an inextricable mixture of fact and hypothesis is represented in this address, but if it shall result in suggesting experiments which will throw any light on the nature of the attractions between atoms or molecules that find expression in the production of crystals, its purpose will be accomplished. NOTES 1 Presidential address, presented at the fourth annual meeting of the Mineralogical Society of America, Washington, D. C., December 29, 1923. The inspiration for this paper has come from the work of my honored teacher, Professor Victor Goldschmidt, on the significance of force-lines perpendicular to faces. 2 See M. Volmer, Z. physik. Chem., 102, 267-275, 1922; some of Dr. Volmer's views have been made use of in working up the present paper, and my indebtedness to him is hereby acknowledged. 3 The work of the late Professor Fedorov has been freely drawn upon in the preparation of the present paper. 4 Professor P. Niggli and his colleagues have, however, put forward suggestions along this line, which have aided the present writer in formulating his ideas. 5 Thanks are hereby extended to Mr. George L. Keenan of the Bureau of Chemistry for his kindness in preparing the slides and photographs of sodium chloride. [var:'startyear'='1924'] [Include:'footer.htm'] |